This afternoon, I had a little tête-à-tête in my office with a buddy who was a math major turned TCS enthusiast.
Me: I’m scratching my head here. The author of this paper I’m reading just casually skipped another proof. I’m starting to think they might be off track.
Him: Whose paper is it? Let me see… Hmm, well, it’s very unlikely that it’s him who is making a mistake Ariana…
Me: Fine… But let me give it a shot. Remember that time I went on a rubbishing about authors skipping proofs and trivializing everything. Yeah I know that he probably wasn’t intentionally trying to baffle readers by skipping steps, but this feels a tad too speedy for me. But it’s tolerable, I’ve seen far worse.
Him: Perhaps he believes it’s already detailed enough in terms of math deductions…
Anyway. I managed to crack it (after enduring the most dreadful gym session). It’s an analysis of a tweaked greedy algorithm for an auction designed to cater to value maximizers (compared to common utility maximizers), who also comes with budget and RoS constraints.
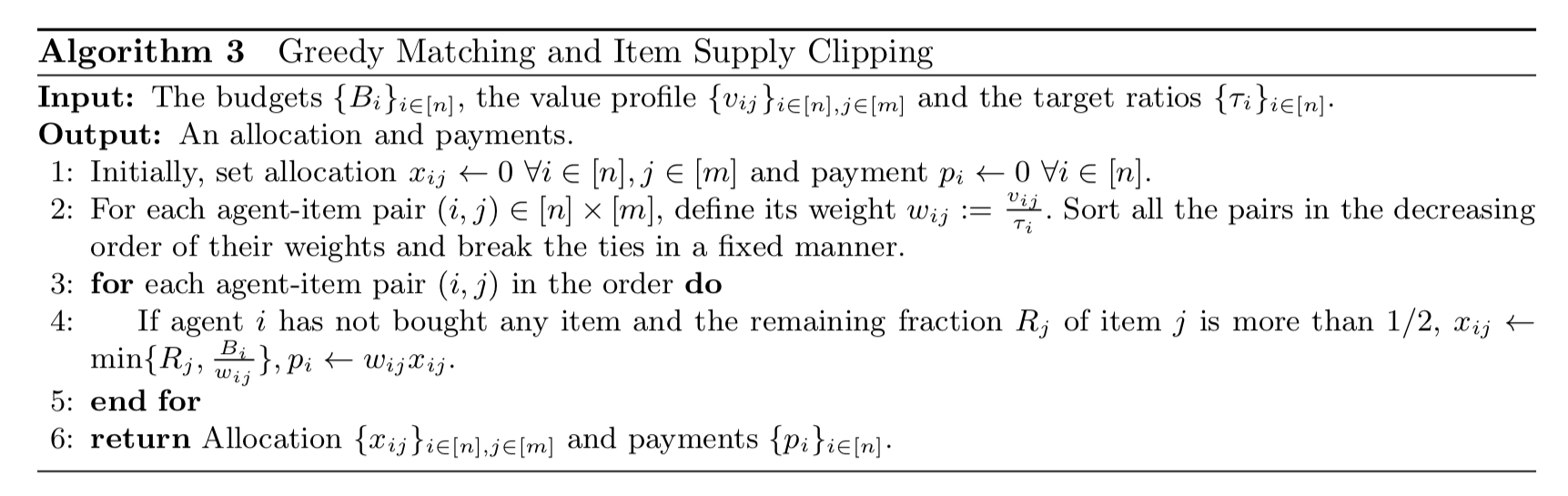
The paper establishes that Algorithm 3 has an approximation ratio of $1/6$ w.r.t. the optimal allocation $\mathbf x$ that maximizes revenue. Denote $\mathbf x$ and $\mathbf x^*$ as the allocations for Algorithm 3 and the optimal solution, respectively. There’s a step that needs to prove $$ \sum_{i\in A}x_{ij}^*w_{ij}\le \sum_{i\in [n]}x_{ij}^*w_{ij}\le 2W_j(\mathbf x) $$ where $W_j(x):=\sum_{i\in [n]} p_{ij}$ is the revenue earned from object $j$, and $A\subset [n]$ is, well, some subset of agent set $[n]$. The author just claimed the above “due to the greedy property of the algorithm”. Cause the algorithm itself is not a classical greedy, so I thought “hmmm, not necessarily does it hold”. (all without a hint of complaint!) But it turns out that this is just trivial, since $w_{ij}$ is chosen greedily, and some property of $A$ guarantees that $\sum_i x_{ij}\ge \frac12$, the result holds naturally.
While I’m revisiting the paper tonight, it seems that the author actually spelled out this idea clearly in the paper. Sorry… my bad. Overall it’s a smooth paper to read. The math is elegant and, I would dare say, beautiful. There are totally 10 algorithms in it and I managed to follow til no.5. Count as a progress for me.
Btw, in case anyone’s curious, here’s the paper —> Lu, Pinyan, Chenyang Xu, and Ruilong Zhang. “Auction Design for Value Maximizers with Budget and Return-on-spend Constraints.” arXiv preprint arXiv:2307.04302 (2023).